proofofconcept

The Physics Derivation Graph focuses on the following:

• Claim: a finite static directed graph exists which describes all of mathematical Physics.
• Claim: the graph representation is machine parsable
• Claim: validity of (some) derivation steps in the graph can be checked using a computer algebra system
• Claim: correctness of (some) derivation steps in the graph can be proven formally

As of May 2024 claims 1, 2, and 3 are demonsrated; work on 4 is planned.

Caveats:

• I’m not aware of currently available computer algebra systems are capable of validating all of mathematical Physics.
• The graph relies on manually checking the equivalence of expressions represented using Latex, Sympy, and Lean.

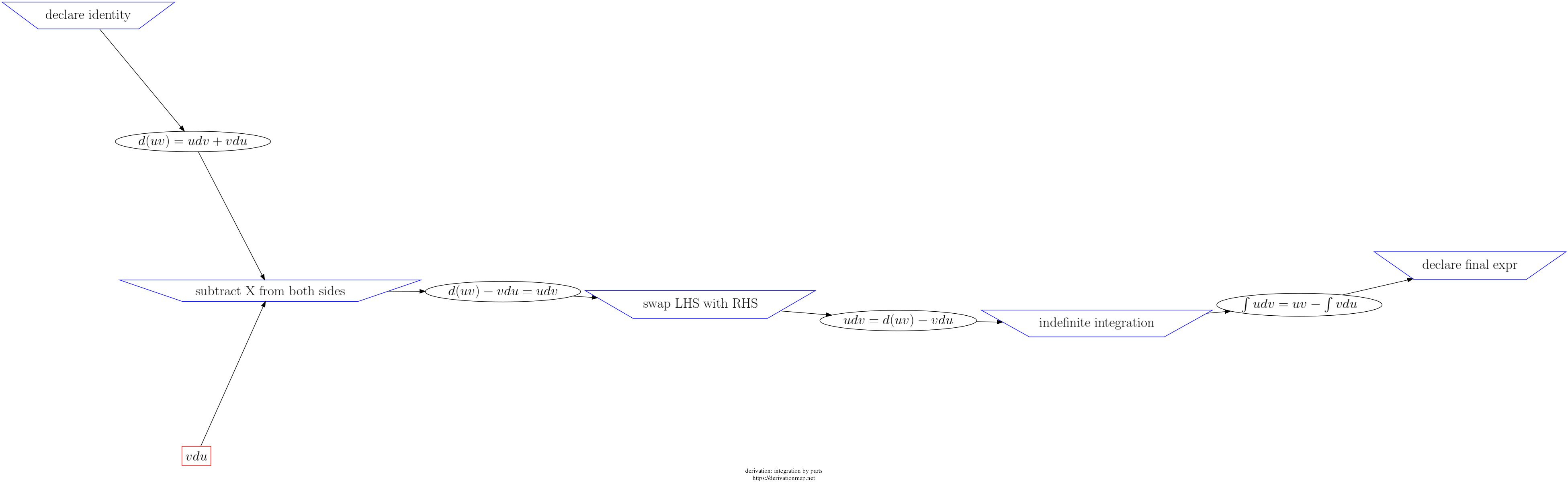
A novel result of this work has been the enumeration of inference rules used in mathematical Physics.

Goals / Objectives

Initial Objectives

• Create a framework capable of describing all mathematics needed for physics derivations. PDG uses Latex as the syntax in the framework because Latex is how I think of equations. However, Latex is insufficient for processing by computer algebra systems. Status: proof of concept exists
• Create machine-readable databases which use the above framework to capture the mathematical derivations in physics. To hold the content of the databases I’m using custom XML. Status: proof of concept exists
• Create graphical representation of relations content in the databases. PDF uses d3js (previously GraphViz) to render the visualization. Status: proof of concept works
• Use a computer algebra system to verify the relations in the databases. PDG uses SymPy as the CAS. Status: proof-of-concept completed in 2020
• Create a web browser-based viewing of the generated graph. HTML5 seems capable. Status: proof-of-concept completed in 2020
• Create a web browser-based graph input tool. Status: proof-of-concept completed in 2020

The proof-of-concept for all the initial objectives were completed during the Covid-19 lockdown (summer of 2020).

Current unmet objectives

• Use a property graph (e.g., Neo4j) and enable users to query the graph. Status: underway as of May 2024. See <
• Adapt visualizations to the new property graph database
• Adding content manually is burdensome. Find automations
• Eliminate website errors
• Enact testing of the backend software and the web UI.
  • See https://www.youtube.com/watch?v=RLKW7ZMJOf4 and https://flask.palletsprojects.com/en/3.0.x/testing/ which use PyTest
  • See https://www.selenium.dev/selenium-ide/

Licensing

Creative Commons Attribution 4.0 International License

a small derivation

Aspirational web interface:

http://www.apcjones.com/arrows/ and https://arrows.app/

Contributing

Before submitting code via a pull request, please open an issue (feature or bug) and describe your question or intent.

Python code is formatted using Black.

This site is open source. Improve this page.